Maths Tuition
GCSE Mathematics
At Infinity Education, our aim is to support your child as a learner of mathematics to develop their confidence, understanding and resilience.
Our GCSE Mathematics programme will cover the following topics:
Working with Number
Ratio & Proportion
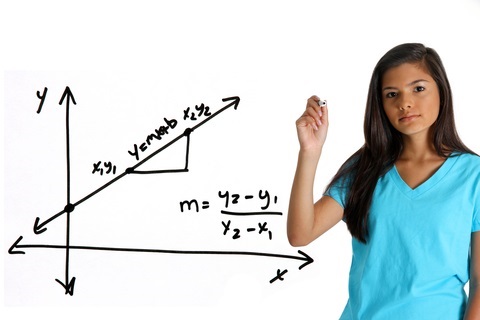
Algebra
Probability
Handling data
Space, Shape and Measures
Before lessons are delivered to the students, parent will receive an email with the tasks for the lesson to print off. All the lessons we deliver are designed to embed knowledge, strengthen conceptual understanding and help each student to comprehend exam questions. Our aim is to develop every student’s application and problem solving skills whilst developing their fluency in Mathematics.
We will frequently assess your child using past exam paper resources to identify weaknesses and gaps in their knowledge.
We offer online tutoring for GCSE Higher and GCSE Foundation for the following exam boards:
AQA, Edexcel, OCR
We also offer tuition for iGCSE Mathematics.
Study Programme
A typical 25 week programme of study for a Year 11 student would be as follows:
| Weeks 1-6 | NUMBER TOPICS |
| Weeks 7-19 | RATION & PROPORTION TOPICS |
| Weeks 10-15 | ALGEBRA TOPICS |
| Week 16-18 | PROBABILITY TOPICS |
| Week 19-21 | DATA HANDLING TOPICS |
| Weeks 22-25 | SHAPE, SPACE AND MEASURES TOPICS |
A-Level Mathematics
Our team of Mathematics tutors are very experienced A-level specialists. Building upon prior knowledge, we will guide them through the core Mathematics, mechanics and statistics ensuring that students have a strong conceptual understanding of each topic and are able to apply their understanding to a range of exam style problems.
Many students enjoy Mathematics but find some aspects of the A-level course incredibly challenging. Many concepts are completely new to the students as they cannot rely on elements of student’s prior learning from their GCSE studies. At Infinity Education, we will support your child through their A-level studies to ensure they are fully prepared for the A-level external examinations.
We will frequently assess your child to identify weaknesses and gaps in their knowledge as well as all their progress.
Study Programme
Our A-level Mathematics tuition programme will cover many of the following topics depending on learning needs of students:
Core Mathematics
Differentiation of polynomial functions, Finding tangents and normals, Stationary points, Integration of polynomial functions, Area under curves, Exponential functions and ex, Logarithms, Natural logarithms, Logarithms and non-linear data, Proof by contradiction, Standard proofs, Partial Fractions, Algebraic division, The modulus function, Modulus transformations, Functions and mappings, Arithmetic sequences/series, Geometric sequences/series, Sigma notation, Recurrence relations, Binomial expansion with negative/fractional powers, Radians and applications, Small angle approximations, Reciprocal trigonometric functions, Pythagorean identities, Inverse trig functions, Addition formulae, Double angle formulae, Trigonometric equations, Harmonic identities, Parametric equations, Derivatives of sin(x) & cos(x) from first principles, Derivatives of standard functions, The chain rule, The product rule, The quotient rule, Parametric differentiation, Implicit differentiation, Concave/convex functions and points of inflection, Rates of change, Locating roots, Iteration, The Newton-Raphson method, Integrating standard functions, Integrating f(ax+b), Integration using trig identities, Integration by substitution, Integration by parts, Integration using partial fractions, Integration by inspection/recognition, Parametric integration, The trapezium rule, Solving differential equations, 3D coordinates and vectors, Geometric vector problems.
Mechanics
Use of vectors, Connected particles, Pulleys, Variable acceleration in 1D, Moments, Resolving forces (inclined planes), Friction, Projectiles, Statics, Rigid bodies/moments, Dynamics, Vectors in kinematics, Variable acceleration in 2D.
